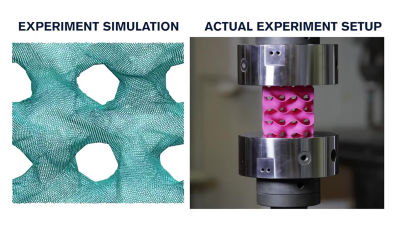
A new shape that exists in nature, the 'soft cell,' has been discovered, a shape that has two acute angles in two dimensions and no acute angles in three dimensions.
A research team from the Budapest University of Technology and Economics and the University of Oxford has discovered a new shape cluster that exists in nature: ' soft cells .' Soft cells are found in a variety of places, such as the cross section of an onion, muscle fibers, and the inside of a nautilus shell, and have the characteristic that they have two acute angles in two-dimensional space and no acute angles in three-dimensional space.
Soft cells and the geometry of seashells | PNAS Nexus | Oxford Academic
What Do Onion Skins and Zebra Strips Have in Common? New Geometric Shapes Identified by BME Mathematicians | BME central site
https://www.bme.hu/en/news/240910/bme-epk-research-soft-cells-discovery-publication
Mathematicians discover new universal class of shapes to explain complex biological forms | University of Oxford
https://www.ox.ac.uk/news/2024-09-12-mathematicians-discover-new-universal-class-shapes-explain-complex-biological-forms
There is a field of mathematics that studies shapes that fill space without gaps. Generally, the objects of study in this field are shapes that are made up of straight lines and multiple acute angles, such as triangles and polygons, but these shapes are not often found in nature.
The research team sought to mathematically explain the 'shapes without straight lines or sharp angles' formed by objects in the natural world, and discovered that many objects in nature are made up of a new mathematical shape called a 'soft cell.'
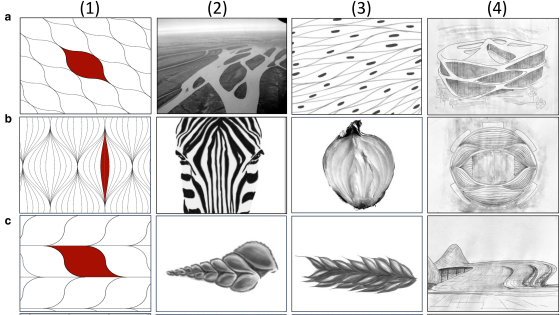
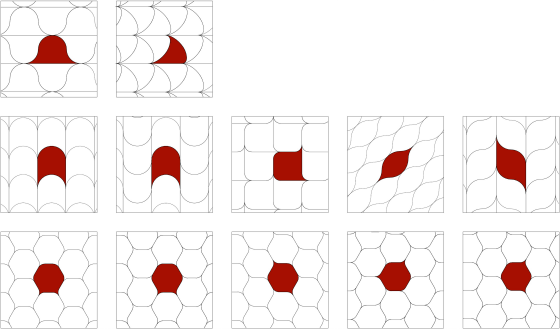
Soft cells are expressed in two-dimensional space as 'figures consisting of two corners and curves.' Below is an example of a tile structure made up of soft cells. The top row is a tile structure of 'soft cells resembling triangles,' the middle row is a tile structure of 'soft cells resembling squares,' and the bottom row is a tile structure of 'soft cells resembling hexagons.'
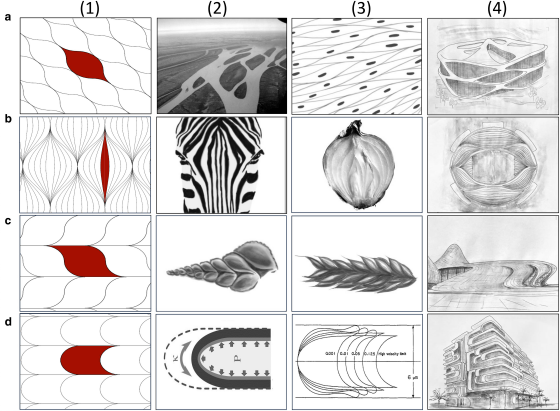
Below are examples of soft cells appearing in nature. Soft cells are observed in 'rivers,' 'zebra stripes,' 'shell cross-sections,' 'geometric models of algae tips,' 'muscle fibers,' 'onion cross-sections,' 'wheat,' and 'cross-sections of blood cells.' The rightmost column also shows that soft cells appear in the design of
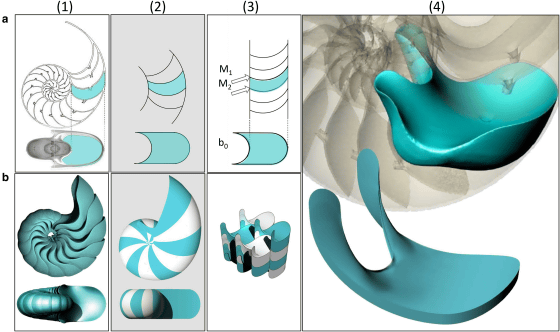
In three-dimensional space, a soft cell appears as a shape with no sharp angles. The research team presents the nautilus shell as an example of a soft cell in three-dimensional space.
'In many cases, the potential applications of new geometric structures become clear many years after their discovery,' said Professor Gabor Domokos , a member of the research team. 'Soft cells have already been shown to be applicable to mathematical, biological and architectural problems, but they may prove to be scientifically useful in other fields in the future.' Professor Alain Goriely , also a member of the research team, commented, 'Not only does nature abhor vacuums, it also seems to abhor sharp angles.'
Related Posts:
in Science, Posted by log1o_hf