Why do prime numbers form a spiral?

Prime numbers may not seem like a regular pattern at first glance, but when linked together from any point of view, they can appear to form a spiral. Mathematician Grant Sanderson explains how this property relates to the fascinating world of mathematics.
3Blue1Brown - Why do prime numbers make these spirals?
Consider placing points on a two-dimensional plane. In the image below, point (1,1) is located at an angle of 1 radian and a distance of 1 from the origin. Similarly, place points (2,2), (3,3), and (4,4).
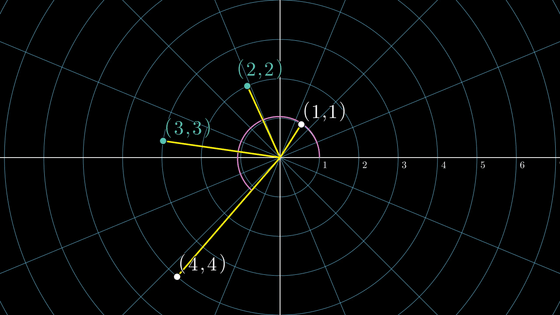
If you continue to add dots in this way, the shape will gradually expand into a spiral and form a figure called the 'Archimedes spiral.'
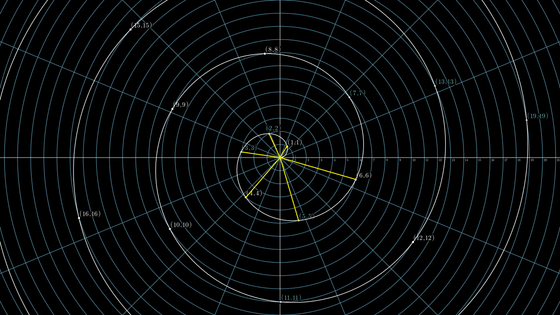
If you remove all the non-prime numbers from this shape, it looks like this. The holes are the prime numbers, but they don't seem to be regular at all.
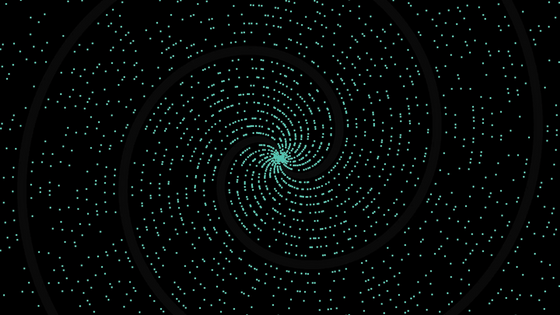
However, if you look at this on a larger scale, a different pattern emerges: the holes are still there, but the beams of light appear to be split into groups of four. Play the video below to see how the four beams are highlighted:
If you look more closely, you'll see that the tiny spiral is made up of 20 lines, but on a larger scale it appears to be made up of a total of 280 lines.
However, this pattern is not limited to prime numbers; similar lines appear when picking out integers alone. However, it is a mystery why this pattern arises, and if we focus only on prime numbers, we arrive at one of the most important theorems regarding the distribution of prime numbers , the Dirichlet theorem .
Looking more deeply into the spiral, we see a regular sequence of numbers forming a line: one line is a multiple of 6 (6k), the next is 6k+2, the next is 6k+3, and so on until we come to the number 6.
This is because, since the angle is defined as 1 radian, rotating 6 radians is almost a full rotation. Since 6 radians is less than 360 degrees (about 344 degrees), the points are arranged in a spiral pattern.
Sanderson explains the value of being exposed to the fun of math in this way: 'Sure, studying in a textbook or a course allows you to learn important facts in a more focused way and with far fewer boring dead ends. But there's something special about rediscovering these topics for yourself. When you learn them by effectively reinventing Euler's tossant function before it's been defined, or wondering about rational approximations before learning about continued fractions, or seriously exploring how primes are divided between remainder classes before you've even heard of Dirichlet, you start to see them as friendly friends rather than as imposed definitions.'
Related Posts:
in Posted by log1p_kr