A new kind of "symmetric polyhedron" structure is discovered for the first time in 400 years

A solid surrounded by four or more planes is called "polyhedron"Especially, all sides are composed of congruent regular polygons"Regular polyhedron"Is a solid with the most beautiful symmetry, it is known that there are only five types such as a tetrahedron. As a variant of this regular polyhedron, polyhedrons with symmetry have been invented by relaxing requirements, but in fact for the first time in 400 years new symmetry polyhedra were invented by American mathematicians.

After 400 years, mathematicians find a new class of solid shapes
http://theconversation.com/after-400-years-mathematicians-find-a-new-class-of-solid-shapes-23217

A "regular polyhedron" (commonly called "Plato's solid") is a solid whose all faces are composed of congruent regular polygons and in which the same number of faces are in contact at all vertices, a regular tetrahedron, a regular hexahedron (cube) It is proved that there are only five kinds of face, regular dodecahedron, regular icosahedron.


After that, by relaxing the condition that all aspects are congruent, "Semi-regular polyhedron"(Commonly known as Archimedes' stereo) was invented. Known as "soccer ball type"Truncated icosahedron"Is especially famous.


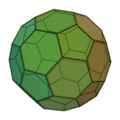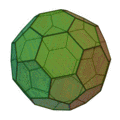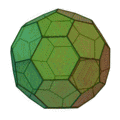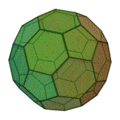
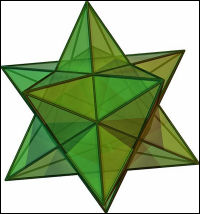
Furthermore, by relaxing the convexity of the regular polyhedron, as a new regular polyhedron, "Star shaped regular polyhedron"(Commonly known as Kepler · Poinso's stereoscopic) 2 kinds as a mathematician of GermanyJohannes KeplerDiscover. After that, two kinds of star shaped regular polyhedrons were discovered and it became four kinds in total, but Kepler discovered the star shaped regular polyhedron about 400 years ago, after that, symmetrical polyhedron was discovered It was not done.

Star dodecahedron of star shaped regular polyhedron.


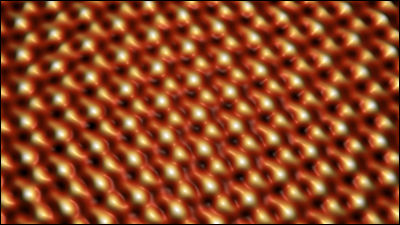
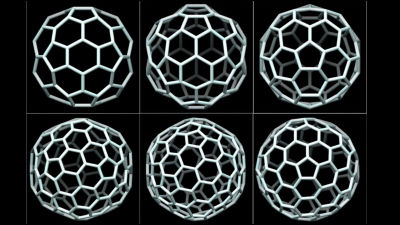
Dr. Stan Shay of the University of California is interested in the structure of protein called clathrin in the process of studying the retina of the eye and mathematically analyzes its three-dimensional structure, Dr. Michael Goldberg of the twentieth century mathematician I will find the polyhedron (Goldberg's solid) that I invented.


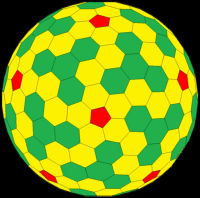
Goldberg's solid is not strictly considered as a polyhedron, since the plane that makes up the solid is not a plane. However, Dr. Shayne, inspired by Goldberg's solid, relaxes the requirement of "the plane is a plane", which is a prerequisite for the polyhedral structure, and allows a "twisted surface structure" which is not a perfect plane So we succeeded in devising a solid which should be said to be "symmetry / quasi-polyhedron" with new symmetry.

This is a new symmetric three-dimensional structure devised by Dr. Shayne and its research team. Although the hexagonal surface making up the solid has a twisted structure, it shows that it depicts beautiful symmetry. According to Dr. Shayne, there is a possibility that innumerable shapes with symmetry can be created by adjusting the degree of twist and increasing the number of faces.


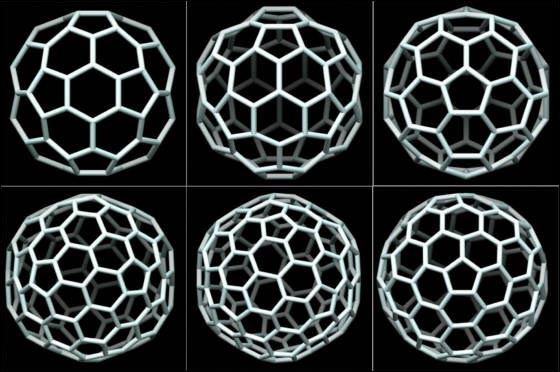
The new symmetrical polyhedron devised this time is sufficiently conceivable as "the face is not flat in the first place, even polyhedrons are not even polyhedral". However, Dr. David Kraven of Birmingham University said that whether this discovery is recognized as a new symmetrical polyhedron for the first time in 400 years depends on "whether we can tolerate the twisted face tolerance" Even though I am highly appreciating the point that it can achieve superb symmetry by giving a specific twist condition.

In general, such mathematical discoveries are rarely applied and utilized technically immediately. However, the three-dimensional structure of "a new symmetric polyhedron" invented by Dr. Shayne is currently considered to be the structure of a specific influenza virus that has not established a fundamental therapeutic method, It is expected that this polyhedral consideration can be applied to grasp and establish therapeutic techniques.