Jun 19, 2024 17:00:00

Indian physicist accidentally discovers new formula for pi while researching string theory

Scientists at the Indian Institute of Science have reported that they have accidentally discovered a new way to express pi (pi) while studying the behavior of high-energy particles.

Phys. Rev. Lett. 132, 221601 (2024) - Field Theory Expansions of String Theory Amplitudes

https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.132.221601

Indian Institute of Science
https://iisc.ac.in/events/iisc-physicists-find-a-new-way-to-represent-pi/

The new formula for Pi was discovered by Aninda Sinha (left) and Arnab Saha (right) of the Centre for High Energy Physics at the Indian Institute of Science.

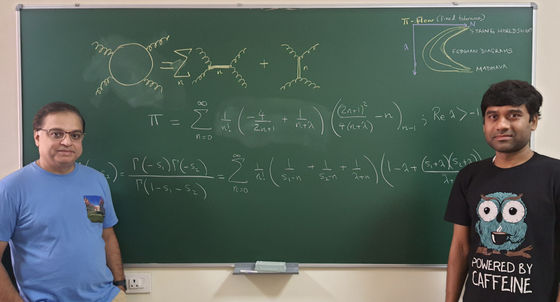
'We were working on developing more accurate, fewer models to study high-energy quantum physics and understand how particles interact,' Sinha said. 'We were excited when we found a new way to look at pi.'

The string theory that Sinha and his colleagues studied is a theoretical framework that treats particles as vibrating 'strings' rather than as points. This theory, also known as 'string theory,' explains that the differences between the elementary particles that make up the natural world are due to the patterns in which the strings vibrate, that is, the vibration modes.

In the study of elementary particles, it is important to understand the interactions of high-energy particles, such as protons colliding in the Large Hadron Collider, as simply as possible, but this is not easy because particles have many degrees of freedom, such as mass, vibration, and motion, that must be taken into account.

Saha, who was working on

an optimization problem to make these calculations as simple as possible, was investigating how to efficiently represent particle interactions. To develop such a model, Saha and Sinha turned to two mathematical tools: the Euler beta function and Feynman diagrams .

The Euler beta function is a mathematical function used to solve problems in many areas of physics and engineering, including machine learning, while the Feynman diagram is a mathematical representation that describes the energy transformation that occurs during the interaction and scattering of two particles.

The model developed by combining these mathematical tools was not only able to explain quantum interactions, but also a series representation of pi.

In mathematics, series are used to express pi in its component parts. If pi is like a dish and the numbers that make up pi are like the ingredients in the dish, then a series can be thought of as a recipe.

It has been difficult to identify the infinite number of combinations that would allow one to quickly find the most accurate value of pi, but the formula that Sinha and his colleagues stumbled upon this time converges quickly to pi, making it useful for calculations such as those analyzing the movement of high-energy particles.

Although Sinha and his colleagues' findings are still theoretical, they may one day be useful in our daily lives. For example, in 1928 Paul Dirac worked on mathematical problems related to the motion of electrons, which later led to the discovery of the positron and the development of positron emission tomography (PET), which is used to screen for diseases and cancer.

'These results may not have immediate applications in everyday life, but they provide a pure joy of learning theory,' Sinha said.